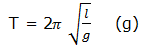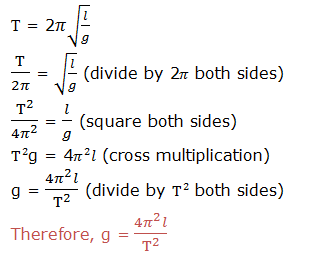Exponents And Radicals - Topic 1: Mathematics Notes Form Two
Exponents
Exponents tell how many times to use a number itself in multiplication. There are different laws that guides in calculations involving exponents. In this chapter we are going to see how these laws are used.
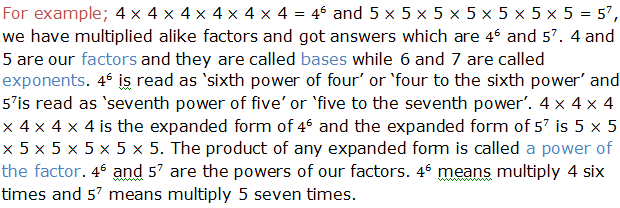


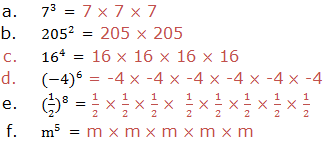
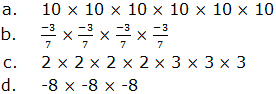
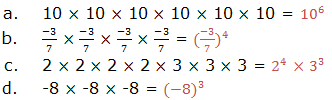
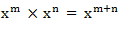
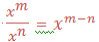
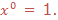
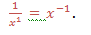
Verification of the Laws of Exponents
Verify the laws of exponents
First law: Multiplication of positive integral exponent
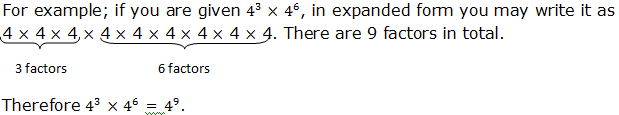
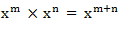
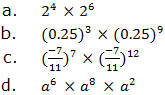

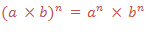
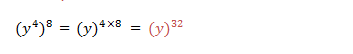
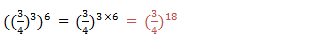
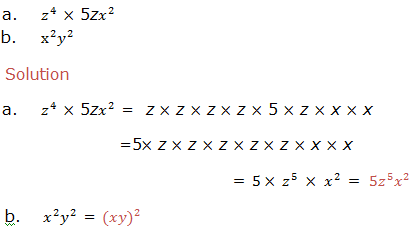

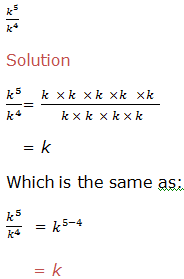
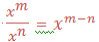
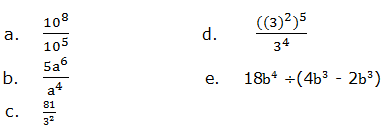

Therefore if x is any real number not equal to zero, then X0 = 1,Note that 00is undefined (not defined).
Fourth law: Negative integral exponents


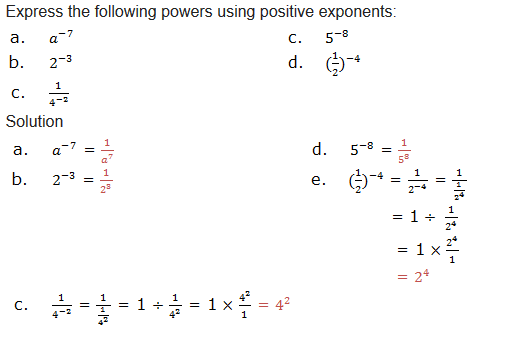


- 169
- 81
- 10,000
- 625

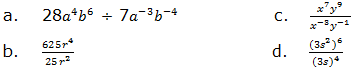

- Base 4
- Base 8
- Base 2
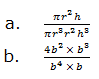


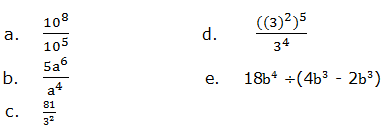

Radicals
Radicals are opposite of exponents. For example when we raise 2 by 2 we get 4 but taking square root of 4 we get 2. The same way we can raise the number using any number is the same way we can have the root of that number.
For example, square root, Cube root, fourth root, fifth roots and so on. We can simplify radicals if the number has factor with root, but if the number has factors with no root then it is in its simplest form. In this chapter we are going to learn how to find the roots of the numbers and how to simplify radicals.
Therefore, the nth root of a number is one of the n equal factors of that number. The symbol for nth root isn√ where√is called a radical and n is the index (indicates the root you have to find). If the index is 2, the symbol represents square root of a number and it is simply written as √without the index 2.n√pis expressed in power form as,
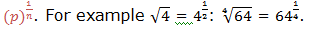


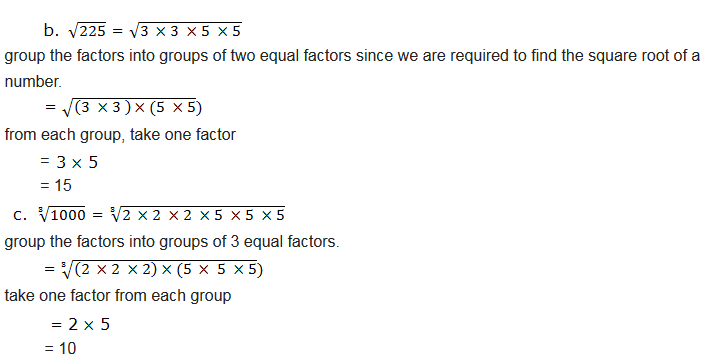
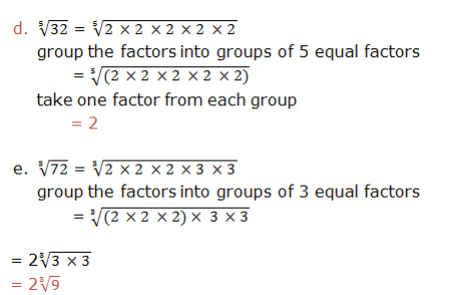
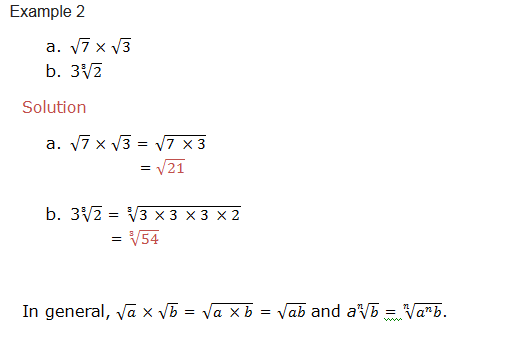
Basic Operations on Radicals
Perform basic operations on radicals
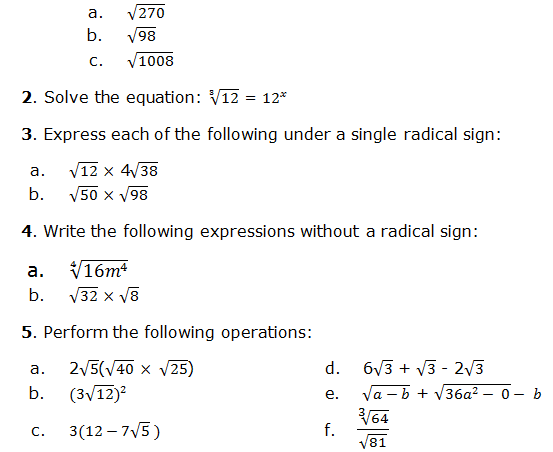
Simplify the following:
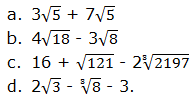



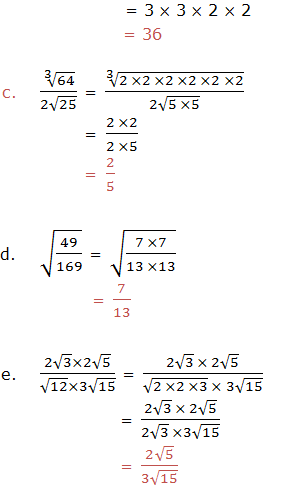
The Denominator
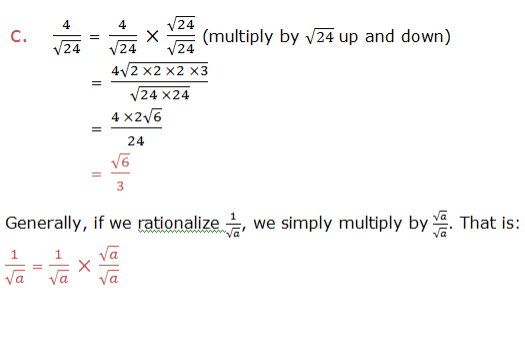
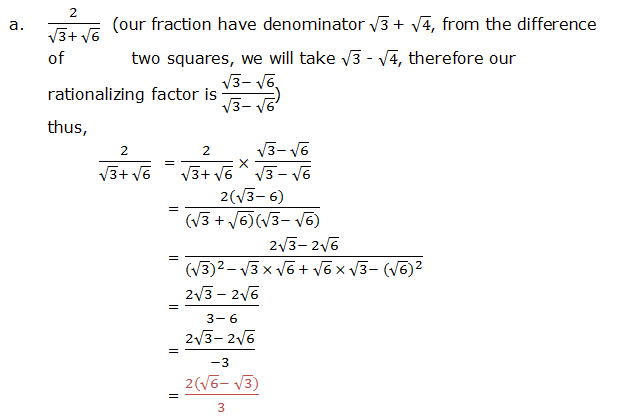
Rationalize the denominator
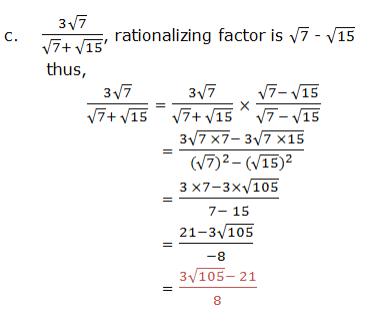
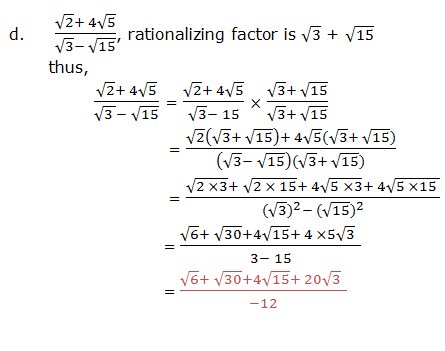
Example 12
Rationalize the denominator of the following expressions:



Square Roots and Cube Roots of Numbers from Mathematical Tables
Read square roots and cube roots of numbers from mathematical tables
If you are to find a square root of a number by using Mathematical table, first estimate the square root by grouping method. We group a given number into groups of two numbers from right. For example; to find a square root of 196 from the table, first we have to group the digits in twos from right i.e. 1 96.
Then estimate the square root of the number in a group on the extreme left. In our example it is 1. The square root of 1 is 1. Because we have two groups, this means that the answer has two digits before the decimal point. Our number is 196, read 1.9 in the table on the extreme left.
From our number, we are remaining with 6, now look at the column labeled 6. Read the number where the row of 1.9 meet the column labeled 6. It meets at 1.400. Therefore the square root of 196 = 14.00 since we said that the answer must have2 digits before decimal points.
Note: If you are given a number with digits more than 4 digits. First, round off the number to four significant figures and then group the digits in twos from right. For example; the number 75678 has five digits. When we round it off into 4 digits we get 75680 and then grouping into two digits we get 7 56 80.
This shows that our answer has 3 digits. We start by estimating the square root of the number to the extreme left, which is 7, the square root of 7 is between 2 and 3. Using the table, along the row 7.5, look at the column labeled 6. Read the answer where the row 7.5 meets the column 6.
Then go to where it is written mean difference and look at the column labeled 8, read the answer where it meets the row 7.5. Take the first answer you got where the row 7.5 met the column 6 and add with the second answer you got where the row 7.5 met the column 8 (mean difference column). The answer you get is the square root of 75680. Which is 275.1
Transposition of Formula
A formula is a rule which is used to calculate one quantity when other quantities are given. Examples of formulas are:

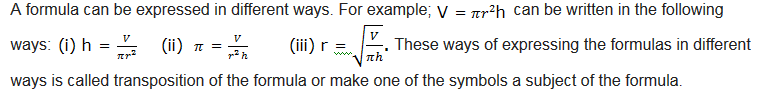

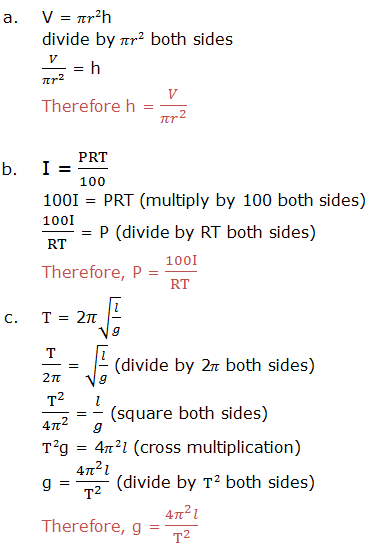
Transposing a Formulae with Square Roots and Square
Transpose a formulae with square roots and square
Make the indicated symbol a subject of the formula: